Wei Li
Gender:Male
Education Level:博士研究生
Alma Mater:中国科学院
Profile
MORE+李伟,北京航空航天大学卓越百人副教授,博士生导师,本科物理专业“固体物理学”主讲教师。2006年本科毕业于北航,2011年博士毕业于中国科学院,2012年-2015年在德国慕尼黑大学从事博士后科研工作,随后回到北航工作。研究方向为凝聚态理论,截止目前在物理学国际学术期刊上发表论文40余篇, 包括 Nature 子刊 1篇(通讯作者), Phys. Rev. X 2篇(通讯作者1篇,共同作者1篇),Phys. Rev. Lett. 2篇(第一作者1篇,共同作者1篇),Phys. Rev. B/E 30篇(一作或通讯作者20篇、含5篇快速通讯,共同作者10篇)等,部分论文可以点击查看arXiv文章列表。
目前承担项目:
北京航空航天大学卓越百人计划,主持,2015.09-2018.08。
北航青年拔尖人才支持计划,主持,2016.10-2020.09。
国家自然科学基金青年项目 “张量网络算法中非阿贝尔对称性的实现及其应用”,主持,2016.01-2018.12。
国家自然科学基金重点项目 “量子多体计算新方法发展及其应用”,子课题负责人,2019.01-2022.12。
国家自然科学基金面上项目 “磁阻挫量子系统的热态张量重正化群研究”,主持,2020.01-2023.12。
科普文章:
【1】量子多体系统的线性张量重正化群方法,《物理》,2012.
【2】有限温度量子多体系统与热态张量网络 ,《物理》,2017.
【3】时空克莱因瓶上的热力学——从二维生物的奇妙旅行到共形量子态的路径积分,《物理》,2018.
国际合作交流:
【1】2017 慕尼黑大学访问学者 (08-11月)
【2】2018 慕尼黑大学访问学者 (02-04月 DFG资助,08-10月)
【3】2020 北航-慕尼黑大学量子物质合作研讨会(2020.08 立项资助 )
主要学术研究成果:
最新进展:三角格子量子伊辛材料TmMgGaO4:二维KT物理与类超流旋子激发 [arXiv:1907.08173 , to appear in Nature Commun.]

H. Li, Y. D. Liao, B.-B. Chen, X.-T. Zeng, X.-L. Sheng, Y. Qi, Z. Y. Meng, Wei Li*, “Kosterlitz-Thouless Melting of Stripe Order in the Triangular Quantum Ising Magnet TmMgGaO4”, arXiv:1907.08173 (2019).
一、发展量子多体系统的有限温度张量重正化群方法
(1)线性张量重正化群方法 [PRL 2011, PRB 2017]

Wei Li, S.-J. Ran, S.-S. Gong, Y. Zhao, B. Xi, F. Ye, and G. Su, “Linearized Tensor Renormalization Group Algorithm for the calculation of Thermodynamics of Quantum Lattice models”, Phys. Rev. Lett. 106, 127202 (2011).
Y.-L. Dong, L. Chen, Y.-J. Liu, and Wei Li*, “Bilayer linearized tensor renormalization group approach for thermal tensor networks”, Phys. Rev. B 95, 144428 (2017).
(2)级数展开热态张量网络方法 [PRB 2017 (R)]
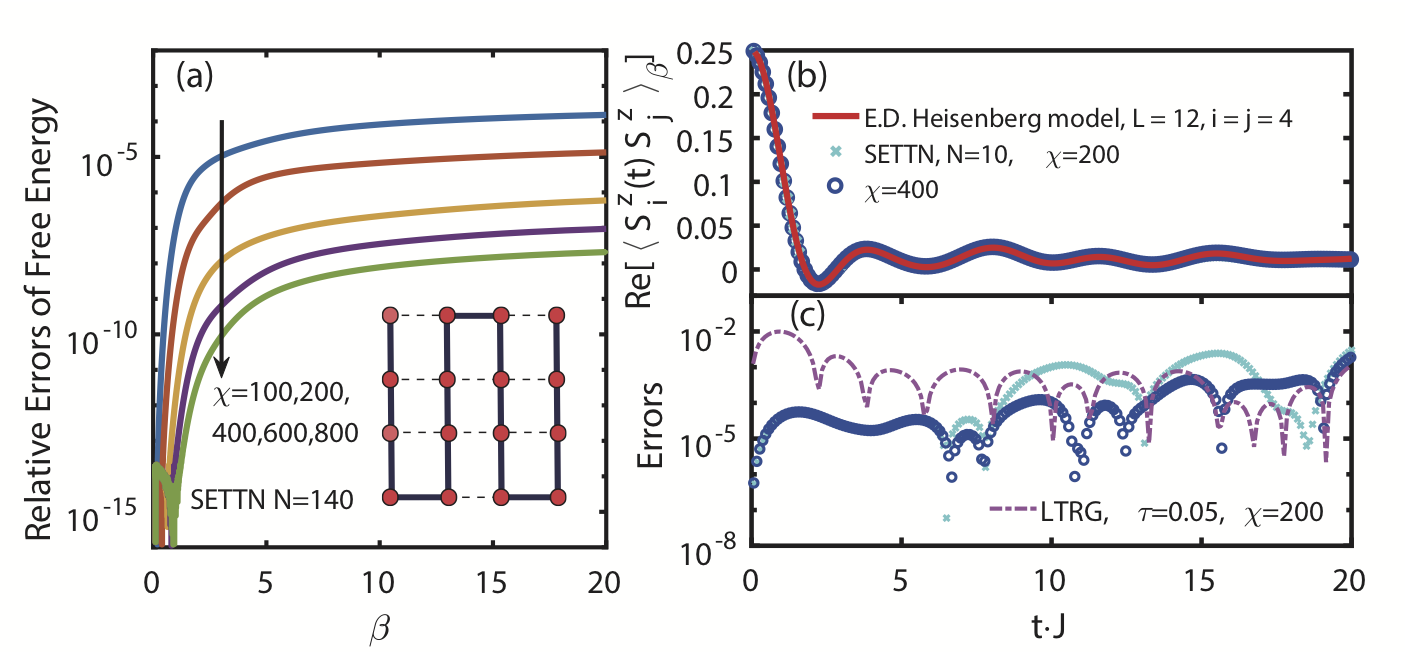
B.-B. Chen, Y.-J. Liu, Z. Chen, and Wei Li*, “Series-expansion thermal tensor network approach for quantum lattice models”, Phys. Rev. B 95, 161104(R) (2017).
(3)指数加速热态张量重正化群 [PRX 2018, PRB 2019]

B.-B. Chen, L. Chen, Z. Chen, Wei Li*, and A. Weichselbaum, “Exponential Thermal Tensor Network Approach for Quantum Lattice Models”, Phys. Rev. X 8, 031082 (2018).
H. Li, B.-B. Chen, Z. Chen, J. von Delft, A. Weichselbaum, and Wei Li*, “Thermal Tensor Renormalization Group simulations of Square-Lattice Quantum Spin Models”, Phys. Rev. B 100, 045110 (2019).
新闻:《物理评论X》刊发北航物理学院微纳物理与应用研究室最新研究成果 (北航主页 ,物理学院主页 ,工信部 )
(4)可微张量重正化群 [arXiv:1912.02780]

B.-B. Chen, Y. Gao, Y.-B. Guo, Y. Liu, H.-H. Zhao, H.-J. Liao, L. Wang, T. Xiang, Wei Li*, and Z. Y. Xie, “Automatic Differentiation for Second Renormalization of Tensor Networks”, arXiv:1912.02780 (2019).
(5) Bethe近似量子多体格点模型的热力学性质计算 [PRB 2019]

Dai-Wei Qu, Wei Li, and Tao Xiang, Thermal Tensor Network Simulations of the Heisenberg Model on the Bethe lattice, Phys. Rev. B 100, 125121 (2019).
二、低维量子磁学与磁性材料
(1)自旋S=1 kagome晶格上的共振AKLT环拓扑态 [PRB 2014, PRB 2015]

Wei Li, S. Yang, M. Cheng, Z.-X. Liu, and H.-H. Tu, "Topology and criticality in resonating AKLT-loop spin liquid state", Phys. Rev. B 89, 174411 (2014).
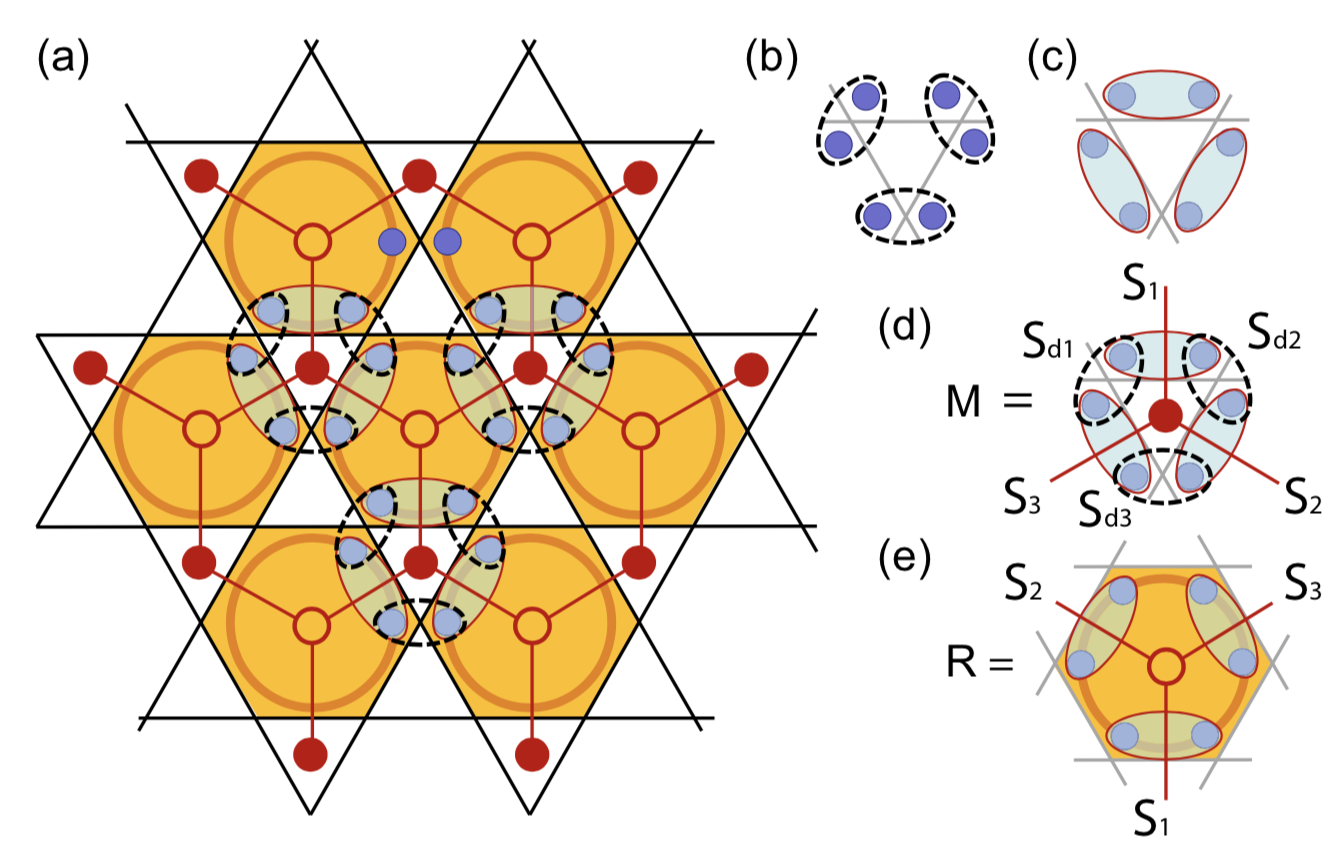
Wei Li, A. Weichselbaum, J. von Delft, and H.-H. Tu, "Hexagon-singlet solid ansatz for the spin-1 kagome antiferromagnet", Phys Rev. B 91, 224414 (2015).
(2)SU(2)张量网络方法与自旋S=1 kagome晶格反铁磁体的共价键晶体态 [PRB 2015(R)]
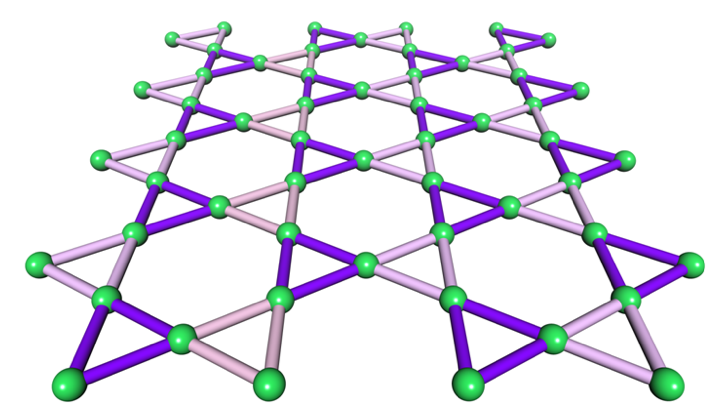
T. Liu, Wei Li*, A. Weichselbaum, J. von Delft, and G. Su,"Simplex valence-bond crystal in the spin-1 kagome Heisenberg antiferromagnet", Phys. Rev. B 91, 060403(R) (2015).
(3)三角格子海森堡反体磁铁中的双温度尺度与Ba8CoNb6O24磁热力学 [PRB 2019(R)]

L. Chen, D.-W. Qu, H. Li, B.-B. Chen, S.-S. Gong, A. Weichselbaum, J. von Delft, and Wei Li*,"Two-temperature scales in the triangular-lattice Heisenberg antiferromagnet", Phys. Rev. B 99, 140404(R) (2019).
三、量子临界普适热力学
(1)共形不变量子临界点的普适热力学 [PRB 2017]

L. Chen, Hao-Xin Wang, Lei Wang, and Wei Li*, “Conformal thermal tensor network and universal entropy on topological manifolds”, Phys. Rev. B 96, 174429 (2017).
W. Tang, L. Chen, Wei Li, X. C. Xie, H.-H. Tu, and L. Wang, "Universal boundary entropies in conformal field theory: A quantum Monte Carlo study", Phys. Rev. B 96, 115136 (2017). [Editor's suggestion]
(2)交叉覆盖流形上的几何与拓扑普适熵 [PRB 2018 (R)]

H.-X. Wang, L. Chen, H. Lin, and Wei Li*, “Topological and geometric universal thermodynamics in conformal field theory”, Phys. Rev. B 97, 220407(R) (2018).
(3)量子自旋链热力学与量子临界增强磁热效应 [Sci. Rep. 2017]

J.-S. Xiang, C. Chen, Wei Li*, X.-L. Sheng, N. Su, Z.-H. Cheng, Q. Chen and Z. Chen, "criticality-Enhanced Magnetocaloric Effect in Quantum Spin Chain Material Copper Nitrate", Scientific Reports 7, 44643 (2017).
四、拓扑物态与量子相变
(1)自旋梯子中的马约拉纳零模 [PRL 2019]

N. J. Robinson, A. Altland, R. Egger, N. M. Gergs, Wei Li, D. Schuricht, A. M. Tsvelik, A. Weichselbaum, and R. M. Konik, “Non-topological Majorana zero modes in inhomogeneous spin ladders”, Phys. Rev. Lett. 122, 027201 (2019).
(2)一维自旋系统的对称保护拓扑序 [PRB 2012, PRB 2013]

G.-H. Liu, Wei Li*, W.-L. You, G.-S. Tian, and G. Su, "Matrix product state and quantum phase transitions in the one-dimensional extended quantum compass model", Phys. Rev. B 85, 184422 (2012).
Wei Li, A. Weichselbaum, and J. von Delft, "Identifying symmetry-protected topological order by entanglement entropy", Phys. Rev. B 88, 245121 (2013).
(3)Parafermion链的量子临界性 [PRB 2015]
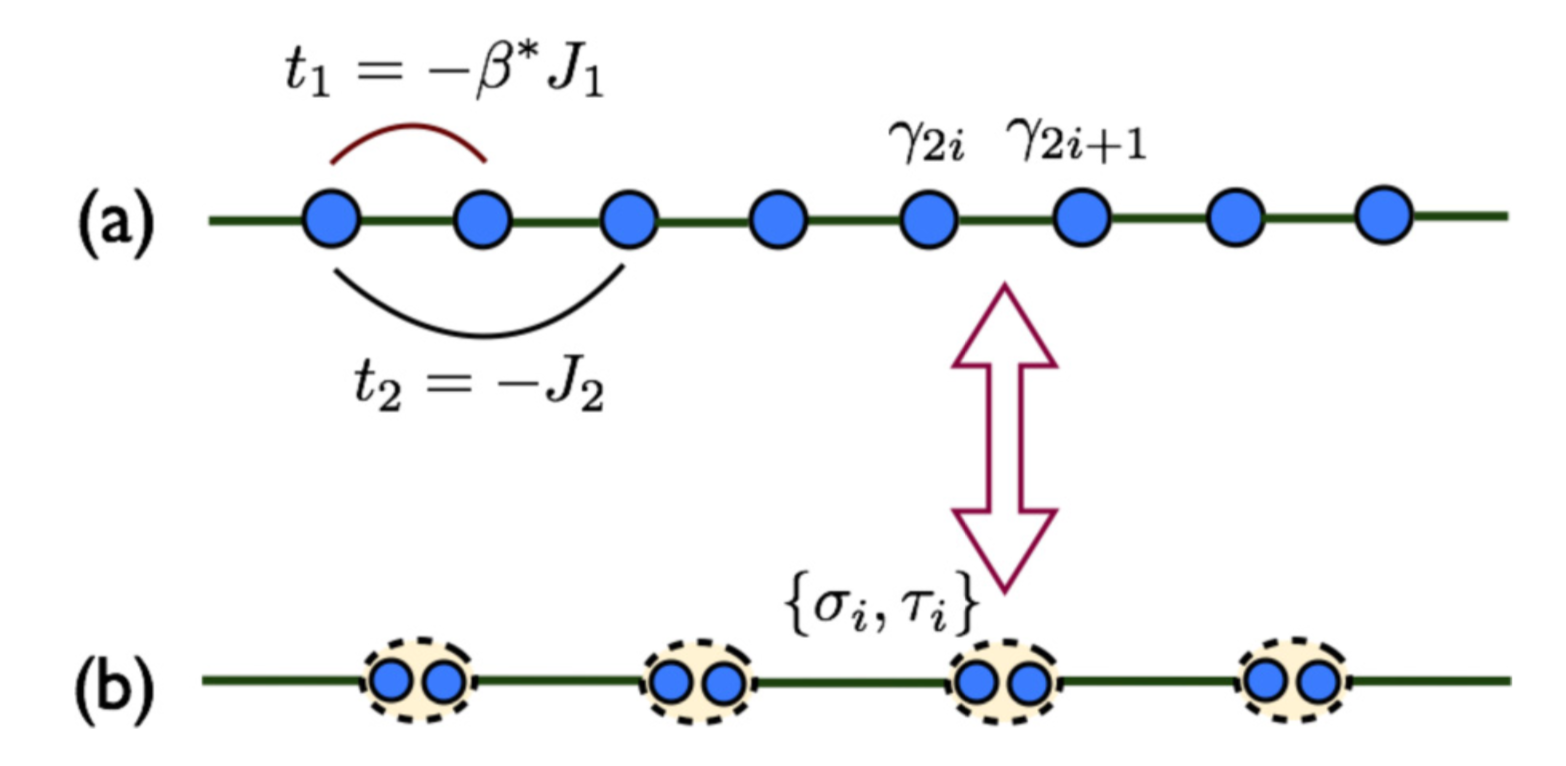
Wei Li, S. Yang, H.-H. Tu, and M. Cheng, “Criticality in translation-invariant parafermion chains”, Phys. Rev. B 91, 115133 (2015).
(4)两维及贝特晶格上自旋系统的量子相变 [PRB 2010, PRB 2012]

Wei Li, S.-S. Gong, Y. Zhao, and G. Su, “Quantum phase transition, O(3) Universality class and phase diagram of Spin-1/2 Heisenberg antiferromagnet on a distorted honeycomb lattice: Tensor renormalization group study”, Phys. Rev. B 81, 184427 (2010).
Wei Li, Jan von Delft, and Tao Xiang, "Efficient simulation of infinite tree tensor network states on the Bethe lattice", Phys. Rev. B 86, 195137 (2012).
五、经典统计模型与相变
(1)阻挫系统的经典相变 [PRB 2010]
Wei Li, S.-S. Gong, Y. Zhao, S.-J. Ran, S. Gao, and G. Su, “Phase transitions and thermodynamics of the two-dimensional Ising model on a distorted Kagome lattice”, Phys. Rev. B 82, 134434 (2010).
(2)统计模型中的拓扑与相变 [PRE 2014, PRE 2015(R)]
S. Li, Wei Li*, and Z. Chen, “Kosterlitz-Thouless transitions and phase diagrams of the interacting monomer-dimer model on a checkerboard lattice”, Phys. Rev. E 90, 052104 (2014).
S. Li, Wei Li*, and Z. Chen, “Topology-driven phase transitions in the classical monomer-dimer-loop model”, Phys. Rev. E 91, 060104(R) (2015).
[1] 2009.3 to 2012.1
中国科学院大学
| 理论物理
| Doctoral degree
| With Certificate of Graduation for Doctorate Study
[2] 2006.9 to 2009.1
北京航空航天大学
| 凝聚态物理
| 物理学硕士
| With Certificate of Graduation for Study as Master's Candidates
[3] 2002.9 to 2006.6
北京航空航天大学
| Physics
| 理学学士
| 本科
[1] 2012.2 to 2015.7
德国慕尼黑大学
| 物理系
| 博士后
